Posts
Wiki Contributions
Speculations on (near) Out-Of-Distribution (OOD) regimes
- [Absence of extractable information] The model can no longer extract any relevant information. Models may behave more and more similarly to their baseline behavior in this regime. Models may learn the heuristic to ignore uninformative data, and this heuristic may generalize pretty far. Publication supporting this regime: Deep Neural Networks Tend To Extrapolate Predictably
- [Extreme information] The model can still extract information, but the features extracted are becoming extreme in value ("extreme" = range never seen during training). Models may keep behaving in the same way as "at the In-Distrubution (ID) border". Models may learn the heuristic that for extreme inputs, you should keep behaving as if you were still in the embedding same direction but still ID.
- [Inner OOD] The model observes a mix of features-values that it never saw during training, but none of these features-values are by themselves OOD. For example, the input is located between two populated planes. Models may learn the heuristic to use a (mixed) policy composed of closest ID behaviors.
- [Far/Disrupting OOD]: This happens in one of the other three regimes when the inputs break the OOD heuristics learned by the model. These can be found by adversarial search or by moving extremely OOD.
- [Fine-Tuning (FT) or Jailbreaking OOD] The inference distribution is OOD of the distribution during the FT. The model then stops using heuristics defined during the FT and starts using those learned during pretraining (the inference is still ID with respect to the pretraining distribution).
If it takes a human 1 month to solve a difficult problem, it seems unlikely that a less capable human who can't solve it within 20 years of effort can still succeed in 40 years
Since the scaling is logarithmic, your example seems to be a strawman.
The real claim debated is more something like:
"If it takes a human 1 month to solve a difficult problem, it seems unlikely that a less capable human who can't solve it within 100 months of effort can still succeed in 10 000 months" And this formulation doesn't seem obviously true.
Ten months later, which papers would you recommend for SOTA explanations of how generalisation works?
From my quick research:
- "Explaining grokking through circuit efficiency" seems great at explaining and describing grokking
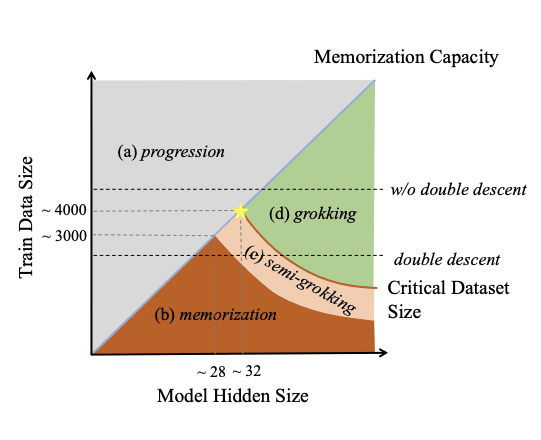
- "Unified View of Grokking, Double Descent and Emergent Abilities: A Comprehensive Study on Algorithm Task" proposes a plausible unified view of grokking and double descent (and a guess at a link with emergent capabilities and multi-task training). I especially like their summary plot:
For information to the readers and author: I am (independently) working on a project about narrowing down the moral values of alien civilizations on the verge of creating an ASI and becoming space-faring. The goal is to inform the prioritization of longtermist interventions.
I will gladly build on your content, which aggregates and beautifully expands several key mechanisms (individual selection ("Darwinian demon"), kin selection/multilevel selection ("Darwinian angel"), filters ("Fragility of Life Hypothesis)) that I use among others (e.g. sequential races, cultural evolution, accelerating growth stages, etc.).
Thanks for the post!
If the following correlations are true, then the opposite may be true (slave morality being better for improving the world through history):
- Improving the world being strongly correlated with economic growth (this is probably less true when X-risk are significant)
- Economic growth being strongly correlated with Entrepreneurship incentives (property rights, autonomy, fairness, meritocracy, low rents)
- Master morality being strongly correlated with acquiring power and thus decreasing the power of others and decreasing their entrepreneurship incentives
Right 👍
So the effects are:
Effects that should increase Anthropic's salaries relative to OpenAI: A) - The pool of AI safety focused candidates is smaller B) - AI safety focused candidates are more motivated
Effects that should decrease Anthropic's salaries relative to OpenAI: C) - AI safety focused candidates should be willing to accept significantly lower wages
New notes: (B) and (C) could cancel each other but that would be a bit suspicious. Still a partial cancellation would make a difference between OpenAI and Anthropic lower and harder to properly observe. (B) May have a small effect, given that hires are already world level talents, it would be weird that they could significantly increase even more their performance by simply being more motivated. I.e. non AI safety focused candidates are also very motivated. The difference in motivation between both groups is possibly not large.
These forecasts are about the order under which functionalities see a jump in their generalization (how far OOD they work well).
By "Generalisable xxx" I meant the form of the functionality xxx that generalize far.
Rambling about Forecasting the order in which functions are learned by NN
Idea:
Using function complexity and their "compoundness" (edit 11 september: these functions seem to be called "composite functions"), we may be able to forecast the order in which algorithms in NN are learned. And we may be able to forecast the temporal ordering of when some functions or behaviours will start generalising strongly.
Rambling:
What happens when training neural networks is similar to the selection of genes in genomes or any reinforcement optimization processes. Compound functions are much harder to learn. You need each part to be independently useful initially to provide enough signal for the compound system to be reinforced.
That means that learning any non-hardcoded algorithms with many variables and multiplicative steps is very difficult.
An important factor in this is the frequency at which an algorithm is useful and to which extent. An algorithm that can be very used in most situations will get much more training signals. The relative strength of the reward signal you get is important because of the noise in the training and because of catastrophic forgetting.
LLMs are not learning complex algorithms yet. They are learning something like a world model because this is used for most tasks and it can be built by first building each part separately and then assembling them.
Regarding building algorithms to exploit this world model, it can be learned later if the algorithm is composed first of very simple algorithms that can be later assembled. An extra difficulty for LLMs to learn algorithms is in situations where heuristics already work very well. In that case, you need to add significant regularisation pushing for simpler circuits. Then you may observe grokking learning and a transition from heuristics to algorithms.
An issue with this reasoning is that heuristics are 1-step algorithms (0 compoundness).
Effects:
- Frequency of reward
- Strength of the additional reward (above the "heuristic baseline")
- Compoundness
Forecasting game:
(WIP, mostly a failure at that point)
Early to generalize well:
World models can be built from simple parts, and are most of the time valuable.
Generalizable algorithm for simple and frequent tasks on which heuristics fail dramatically: ??? (maybe) generating random numbers, ??
Medium to generalize well:
Generalizable deceptive alignment algorithms: They require several components to work. But they are useful for many tasks. The strength of the additional reward is not especially high or low.
Generalizable instrumental convergence algorithms: Same as deceptive alignment.
Generalizable short horizon algorithms: They, by definition, require fewer sequential steps, as such they should be less "compounded" functions and appear sooner.
Late:
Generalizable long horizon algorithms: They, by definition, require more sequential steps, as such they should be more "compounded" functions and appear later.
The latest:
Generalizable long horizon narrow capabilities: They are not frequently reinforced.
(Time spent on this: 45min)
July 6th update:
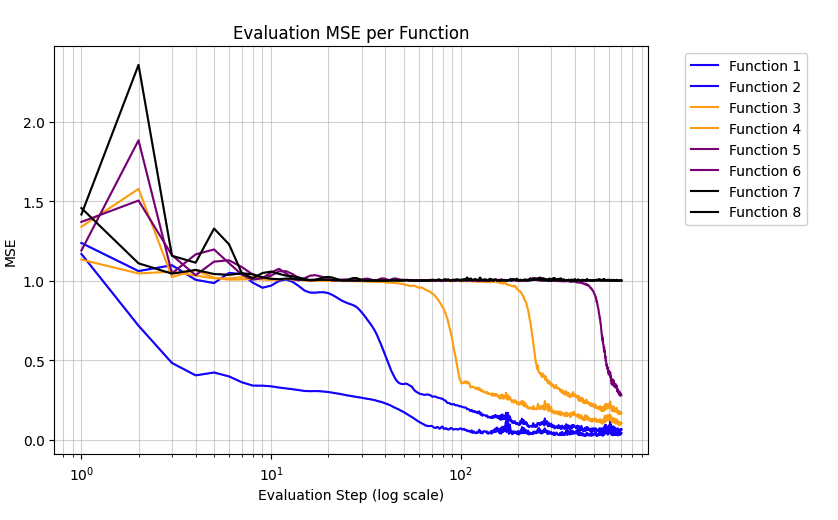
Here is a quick experiment trying to observe the effect of increasing "compoundness" on the ordering of grokking learning different functions: https://colab.research.google.com/drive/1B85mfCkqyQZSl1JGbLr0r5BrAS8LYUr5?usp=sharing
Quick results:
The task is predicting the sign of the product of 1 (function 1) to 8 (function 8) standard normal random variables.
Increasing the compoundness by 2 seems to delay the grokking learning by something like 1 OOM.
Will we get to GPT-5 and GPT-6 soon?
This is a straightforward "follow the trend" model which tries to forecast when GPT-N-equivalent models will be first trained and deployed up to 2030.
Baseline forecast:
GPT-4.7 | GPT-5.3 | GPT-5.8 | GPT-6.3 | |
| Start of training | 2024.4 | 2025.5 | 2026.5 | 2028.5 |
| Deployment | 2025.2 | 2026.8 | 2028 | 2030.2 |
Bullish forecast:
GPT-5 | GPT-5.5 | GPT-6 | GPT-6.5 | |
| Start of training | 2024.4 | 2025 | 2026.5 | 2028.5 |
| Deployment | 2025.2 | 2026.5 | 2028 | 2030 |
FWIW, it predicts roughly similar growth in model size, energy cost and GPU count than described in https://situational-awareness.ai/ while being created the week before this was released.
I spent like 10 hours on this, so I expect to find lingering mistakes in the model.
People may be blind to the fact that improvements from gpt2 to 3 to 4 were both driven by scaling training compute (by 2 OOM between each generation) and (the hidden part) by scaling test compute through long context and CoT (like 1.5-2 OOM between each generations too).
If gpt5 uses just 2 OOM more training compute than gpt4 but the same test compute, then we should not expect "similar" gains, we should expect "half".
O1 may use 2 OOM more test compute than gpt4. So gpt4=>O1+gpt5 could be expected to be similar to gpt3=>gpt4