All of Alok Singh's Comments + Replies
I adjusted H to use heaviside's 1/2 convention, good catch.
https://en.wikipedia.org/wiki/Limiting_density_of_discrete_points

log(infinite number) seems a promising avenue of investigation. also reminiscent of harmonic series--in euler's words-- its sum is the log of an infinite number.
Skipping context sure saved me a lotta time, and plus you gave a nice elab
shoe thrifting is meh for me because foot size
What sort of boots?
From Goldblatt, since {0..H} is internal.

Gonna sleep bc 3 am but will respond later. Also the remark that hyperfinite can mean smaller than a nonstandard natural just seems false, where did you get that idea from?
I used compactness in recent comment reply. Hypernaturals are uncountable because they are bigger than all the nats and so can’t be counted. Whether cardinality of continuum is equivalent to continuum hypothesis
I thought about this since. Bigger is not the right word. Complicated maybe? Like how the unit interval contains non-measurable sub intervals, or a compact set contains non-compact subsets.
Each number gets infinitesimal weight. Which infinitesimal is basically arbitrary.
P v NP: https://en.wikipedia.org/wiki/Generic-case_complexity
iLate reply, but the slicker bit is going in more fully. The appeal of the NSA approach here is axiomatizing it which helps people understand because people already know what numbers are, so 'inf big' is much less of a stretch than going the usual crazy inference depth math has.
This really benefits from a picture. Calling something “a nonstandard number” doesn’t really convey anything about them and a better name I’ll use is “infinitely big”, because they are.
< makes sense because the 2 chains are finite numbers and infinitely big numbers and an infinitely big number is bigger than any finite one because it’s , well, infinite. I can elaborate more technically, but I think trying to develop some numeracy for infinite numbers is a lot like learning about negatives and rationals and complex numbers. Just play with some expression...
"This" is the broken duality phenomenon?
Through stone duality. What about them in particular?
thanks, i think. how'd you find the content?
I think there’s an implicit element of scale or one offness. For buying milk you have multiple samples as to good price. Even if any is contrived, the bulk still capture something real
reminded me of https://en.wikipedia.org/wiki/Winner%27s_curse
lol laffy taffy is too real banana sucks
Train skill of noticing tension and focus on it. Tends to dissolve. No that's not so satisfying but it works. Standing desk can help but it's just not that comfortable for most.
that the functional analysis is mildly helpful for understanding the problem, but the focus of the field doesn't seem to be on anything helpful. VC dimension is the usual thing to poke fun at, but a lot of the work on regularization is also meh
UCSF willed body program, on contract to Merritt College.
(Something that came up yesterday, parens give the particular case.)
Have you spent a lot of time on a skill without a cap? (like math)?
Have you paid money for it? (math tutoring)
How much?
How much have you paid towards a complementary unbounded skill (managing people, voice coaching).
So yeah, between learning another hour of math and a voice coach, both at $~80/hour, is the marginal util of voice coach[1] way lower[2]?.
...I still wonder about the parity prediction these days. I feel like there's something there
Except that you can have a thread just for conversations. It subsumes the chat model.
The point is that such a distribution (uniform on countable infinite set like naturals), is not internal, and therefore external. it'll depend on the specific ultrafilter used under the hood.
for how to use it, see either alain roberts or sylvia wenmackers
One way size goes seems to be:
Limited/finite, actual infinity (countable), potential infinity (uncountable/hyperfinite/compact regions).
On limited and uncountable inputs, we can define a uniform distribution naturally.
A uniform distribution on a countable set, there's no natural way to do that. So in a way, they're "bigger".
the nameless rationalist virtue (void)
Extremely based.
Related: Ends of groups: a nonstandard perspective, Journal of Logic and Analysis, Volume 3:7 (2011), 1-28.
Ends are havens in pursuit games
Differential games lend themselves to a hyperfinite description. You can even have turns. Each player takes an infinitesimal move, then the other goes. A hyperdiscrete approach.
Observing your opponent becomes really important, like in a fight, soccer, or a relationship 😶. I have the intuition that the OODA loop falls out of this.
There's a classic game here where you run from a lion, and the optimum is running along a harmonic spiral since it's infinitely long. What would that look like under this?
A while ago, I saw Dan Savage's film festival. As an intense art student ate 1000 condoms, a thought flashed. Many gears, all ticking. Click. Click. Clock.
And it hit me: every finite commutative group is a product of cyclic groups of prime power order, just like a prime factorization.
I still have no idea how that came in that context.
I was thinking the one corresponding to a unit circle, just the ordinary dot product.
Canon is probably the wrong word in a mathy context.
Also yes the infinitesimal neighborhood of the identity.
Done 🙏
https://link.springer.com/book/10.1007/978-3-642-33149-7
Also includes Feynman path integral and a few other things. Note that you don't even need the full nonstandard theory.
On any finite dim space we have a canon inner product by taking the positive definite one.
Monad is a synonym for infinitesimal neighborhood, common on the literature. Not the category theory monad.
Also hermeneutic lmfao
I'll just say for now. Basically wrapping up "is all this an elaborate simulation designed to convince me that pi = 12"
handy trick inspired by compactification: we can work with completed structures by adding endpoints and taking them away.
example: for a lattice, we can adjoin 0 and 1 as min/max elements by defining a structure where the sentences and are true for all in the original structure, then do what we want, then delete 0 and 1.
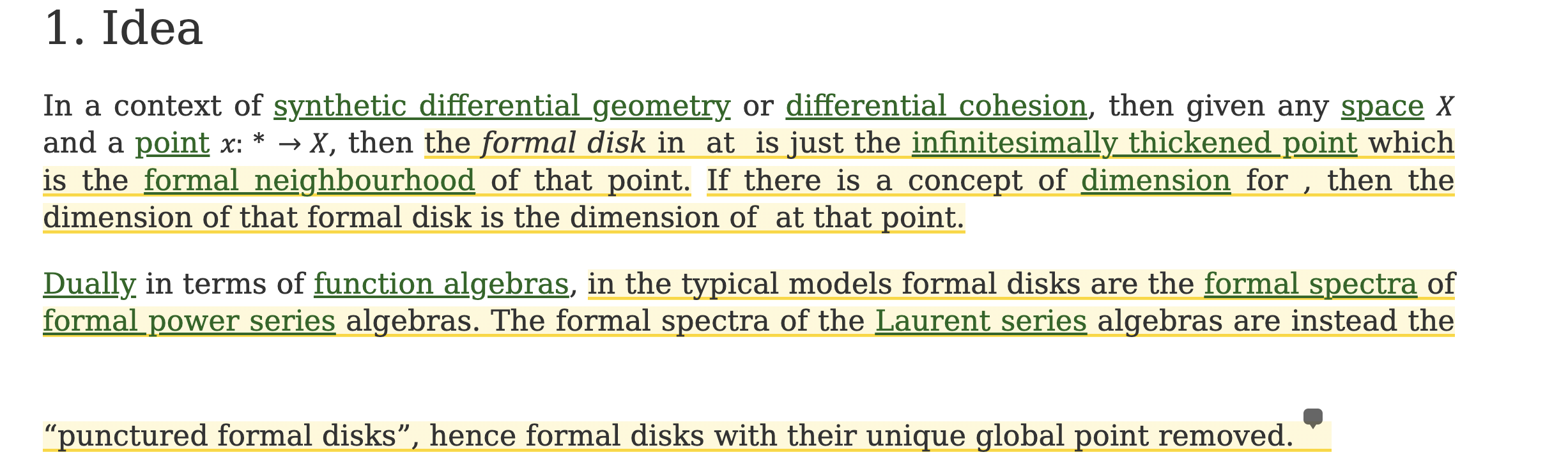
reading https://ncatlab.org/nlab/show/formal+disk convinced me that there's something to my feeling that nonstandard analysis is similar to algebraic geometry.
formal disk ~ infinitesimal neighborhood/halo of a point ~ formal spectrum of power series, which extends prime spectra, one of the main concepts of algebraic geometry. any help here appreciated
Was being deliberately inaccurate here. Hard does mean more than a limited multiplier. Sudden means that there's an appreciable change over an infinitesimal variation aka discontinuous.
Lookup overflow, underflow, and "principle of permanence" in Goldblatt for why I'd do that. Also called overspill and underspill. The basic idea is "as above, so below" except this link is 2 way. Say some internal function has all infinitesimals in its range. Then it must have non infinitesimals too, since the set of all infinitesimals is known to be external, and images of internal functions over internal sets are internal. This is an example of overspill. Infinitesimal behavior has spilled over into the appreciable domain.
Reread this and this is awesome: I didn't think of the case of multiple bars at all. As for the surreal stuff, I've read about them and while the lexicographic ordering is nice, the lack of transfer principle hurts. Are you in the bay?
added some open circles