You are one of the best long-range snipers in the World. You often have to eliminate targets standing one or two miles away and rarely miss. Crucially, this is not because you are better than others at aligning the scope of your rifle with the target’s head before taking the shot. Rather, this is because you are better at accounting for the external factors that will influence the bullet’s direction (e.g., the wind, gravity, etc.). Estimating these external factors is crucial for your success. One day, you are on a roof with binoculars, looking at a building four miles away that you and your team know is filled with terrorists and their innocent children. Your superior is beside you, looking in the same direction. Your mission is to inform your allies closer to the building if you see any movement. After a long wait, the terrorist leader comes out, holding a child close to him. He knows ennemies might be targetting him and would not want to hurt the kid. They are hastily heading towards another building nearby. You grab your radio to inform your allies but your superior stops you and hands you your sniper. “He’s so exposed. This is a golden opportunity for you.” she says. “I reckon we’ve got two minutes before they reach the other building. Do you think you can get him?“. You know that your superior generally accepts risking the lives of innocents but only if there are more bad guys than innocents killed, in expectation. “Absolutely not.” you respond. “We are four miles away! I’ve never taken a shot from this far. NO ONE has ever hit a target from this far. I’m just as likely to hit the kid.” Your superior takes a few seconds to think. “You always say that where the bullet ends up is the result of an equation: ‘where you aim + the external factors’, right?” she says. “Yes,” you reply nervously “And I have no idea how to account for the external factors from that far. There are so many different wind layers between us and the target. The Earth’s rotation and the spin drift of the bullet matter also a hell of a lot from this distance. I would also have to factor in the altitude, humidity, and temperature of the air. I don’t have the faintest idea what this sums up to overall from this far! I’m clueless. I wouldn’t be more likely to get him and not the kid if I were to shoot with my eyes closed.” Your superior turns her head towards you. “Principle of indifference,” she says. “You have no clue how external factors will affect your shot. You don’t know if they tell you to aim more a certain way rather than another. So simply don’t adjust your shot! Aim at this jerk and shoot as if there were no external factors! And you’ll be, in expectation, more likely to hit him than the kid.”
I'm curious whether people agree with the superior. Do you think applying the POI is warranted, here? Any rationale behind why (not)?
EDIT: You can assume the child and the target cover exactly as much hittable surface area if you want, but I'm actually just interested in whether you find the POI argument valid, not in what we think the right strategic call would be if that was a real-life situation. (EDIT 2:) This is just a thought experiment aiming at assessing whether applying POI makes sense in situations of complex cluelessness. No kid is going to get killed in the real world because of your response. :)
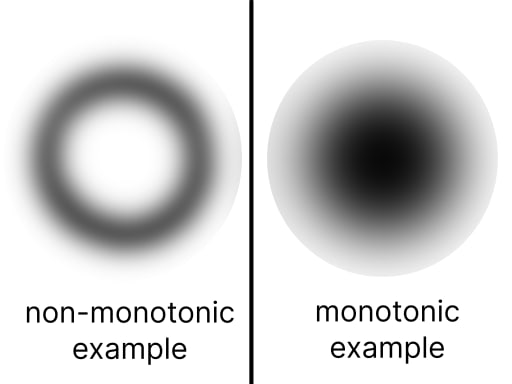
So, as you noted in another comment, this depends on your understanding of the nature of the types of errors individual perturbations are likely to induce. I was automatically guessing many small random perturbations that could be approximated by a random walk, under the assumption that any systematic errors are the kind of thing the sniper could at least mostly adjust for even at extreme range. Which I could be easily convinced is completely false in ways I have no ability to concretely anticipate.
That said, whatever assumptions I make about the kinds of errors at play, I am implicitly mapping out some guessed-at probability density function. I can be convinced it skews left or right, down or up. I can be convinced, and already was, that it falls off at a rate such that if I define it in polar coordinates and integrate over theta that the most likely distance-from-targeted-point is some finite nonzero value. (This kind of reasoning comes up sometimes in statistical mechanics, since systems are often not actually at a/the maxentropy state, but instead within some expected phase-space distance of maxentropy, determined by how quickly density of states changes).
But to convince me that the peak of the probability density function is somewhere other than the origin (the intended target), I think I'd have to be given some specific information about the types of error present that the sniper does not have in the scenario, or which the sniper knows but is somehow still unable to adjust for. Lacking such information, then for decision making purposes, other than "You're almost certainly going to miss" (which I agree with!), it does seem to me that if anyone gets hit, the intended target who also has larger cross-sectional area seems at least a tiny bit more likely.